Despite apparent differences between observations made by observers in relative motion, when in coincidence any and every observer is witness to the same events independent of their relative velocities with respect to each other or the objects upon which the observed events occur. That is called ‘frame independence’. Along with the ‘second postulate’, i.e., the universality of the speed of light in vacuo, it is a major tenet of relativity.
To understand the significance and implications of this aspect of relativity, one must first understand the nature of the frames of reference to which independence is asserted. A frame of reference is associated with an observer; it is a conceptual grid of distances and time intervals between an observed event and the observer him/herself. In Kant’s conception it is a product of pure reason; it is basically how we conceptualize the world around us. It is a world of events, not objects. The reified objects are further creations of our mind that correlates ensembles of events, concluding with physical entities and mechanisms (natural phenomena) that produce the events that are observed. This reality of our conclusions has a firm basis in logic; it is a physical world we strive to understand, but it is not what is directly observed. Relativity is a logical theory that relates the observations, that are necessarily different because they have been made by observers in different situations. Instinctively we believe (for good reason) that the universe observed by any observer in any dynamical state must be the same as that observed by any other.
If an atom, at some location and time of occurrence in my frame of reference, emits a photon of light and there are no intervening obstructions (the atom and observer exist in a vacuum), the photon will be visible to the observer’s eye sometime later. How much later depend only on the distance of our separation because of the universality of the speed of light. Furthermore, and this is the principle of frame independence, it is of no consequence what the motion of the atom when it emitted the photon. This concept is illustrated at the left of figure 1.
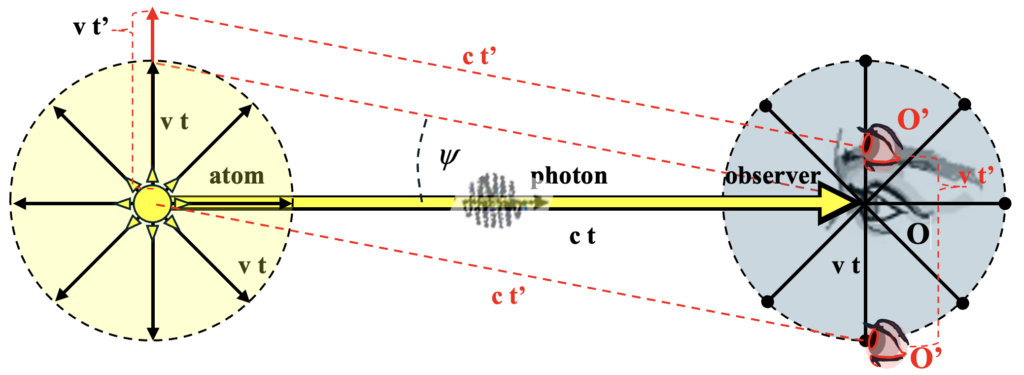
Figure 1: Both aspects of frame independence
This is a consensus view of the tenet, but there is more to it. It’s generally understood among relativity theorists that a frame of reference ‘is’ the observer. There is no error in that view other than that it obscures the simple fact that observation of a photon is itself an event directly similar to an observed event, i.e., the absorption of a photon. It may occur anywhere (or time) within the ‘observer’s’ frame of reference that we conveniently generally translate to the origin at the time zero. Thus, frame independence includes what one might call ‘mutual observability’. At the moment a photon of light struck my retina, that photon must have been visible to any eye that happened to be where my eye was when it detected that particular photon. This is illustrated at the right of figure 1. Other relatively moving observers at a distance v t away from where the observation will take place for O will be coincident when the observation occurs. That is what relativity is all about – what’s good for the goose is good for the gander.
What can get confusing is when an argument that applies to an observed event within one frame of reference is assumed to apply also within other relatively moving frames of reference (observers). This is so confusing and, in fact, the only way to fully comprehend this is by totally embracing the second postulate. Primarily the difference is the relativistic aberration angle but the transit time is not different. Just as there are different observers who observe (detect light), there are different (relatively moving) atoms that host the events of light emission. When there is relative motion, both ends of the transaction cannot involve coincidence. See figure 2.
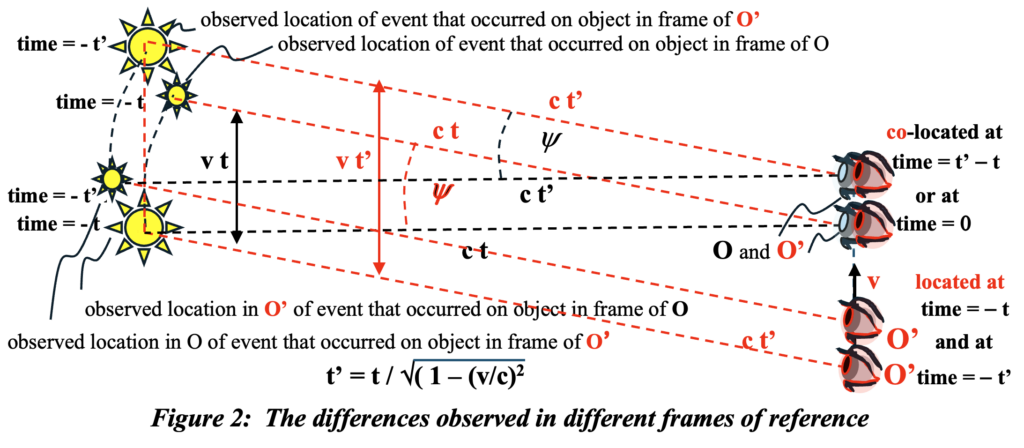
To illustrate this problem, consider the observer O’ at botom right of the figure who is moving at the velocity v relative to O such that they coincide at t = t’ = 0. He will see the angle aberrated through the angle y, but the amount of time for light to get from here to there is not changed because a different observer shows up. So the event that appears at the angle y will have been rotated (rather than ‘skew rotated’) to preserve the time interval. See figure 3.
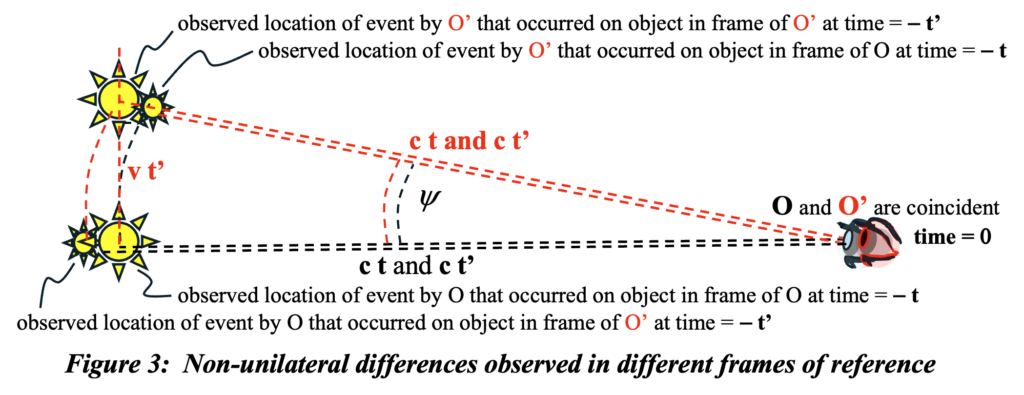
The lesson of frame independence and mutual observability is that there are four observation categories, not just two as has been accepted by the establishment. This is the key reason why it is essential to modify relativity. It must be made compatible with the broader scope of observation. More about relativistic dogma surrounding observation next time.
Leave a Reply